Why upon hearing his arguments I changed my opinion 360 degrees.
Recent comment on the floor of some state legislature

If I take a strap and a wooden ball, glue one end of the strap to the ball and the other end to the floor, turn the ball but in the end leaving the ball oriented as it was, I can then tell by examining the strap whether the ball has been rotated an even or odd number of times. This depends on being able to count the twists in the strap. A string won’t work.

The behavior of electrons is dependent on how many times they have been rotated in this even-odd sense.

If I hold a coin on my finger tips I can rotate it 720 degrees while the coin remains face up, so that my body is back in the original position and my feet did not move. I can not do this for 360 degrees.

These are all the same phenomenon. I don’t know when it was first noted. Dirac became aware of this in his theory of the electron.

I have long known that this could be explained with quaternions. Dirac’s presentation is equivalent, I think. Here is an elementary if not entirely trivial non algebraic proof.

An orientation is a position that a ball can be in when its center is constrained to remain where it began, say at the origin. We will view orientations as a group with a distinguished identity orientation. Rotating a ball 360 degrees about any axis brings it back to the same orientation.

Any orientation leaves some axis fixed. An orientation can be made to correspond continuously to a point inside the ball, along the axis of fixed points. Small rotations correspond to points near the center and large rotations (near 180 degrees) to a point near the surface. In this mapping antipodal points of the ball’s surface are identified and correspond to a 180 degree rotation. A ball with antipodal surface points identified thus has the same topology as the space of orientations.

While we cannot discriminate between two orientations that differ by 360 degrees, we can discriminate between motions that lead to the orientation. A motion is a continuous path thru the space of orientations.

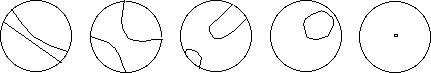
A motion is a map f from the unit interval [0, 1] to the space of orientations and thence via our correspondence to points within the ball. We will consider motions where f(1) = f(0) = the identity orientation. Each such motion brings the ball back to the original orientation. The unit interval thus maps to a loop within the ball which possibly reaches the surface and reenters antipodally some number of times. Two successive surface piercings can cancel by a continuous deformation of the loop and we are thus left with two equivalence classes of loops. A loop with an odd number of piercings corresponds to a motion that leaves the strap twisted. A rotation of 360 degrees can be seen to correspond to a loop that leaves the center, proceeds directly along the axis of rotation to the surface, reenters antipodally and proceeds directly back to the center, thus piercing the surface an odd number of times.

Comments

This situation is much like the use of homotopy to prove that a torus is different from a sphere. Any loop on a sphere can be shrunk to a point whereas some loops on a torus cannot be. Our configuration space is not simply connected for we cannot shrink a path of configurations for a 360 rotation to a point!

Here are some stages in the deformation of a 720 degree rotation into the null motion. All of the action occurs in one plane thru the center of the ball.

Clarification

The above is the crux of the argument but the logic must be elaborated. Rotating the ball thru 360 degrees leads to the same orientation but that motion is not equivalent to the null motion. Two motions are equivalent when one motion can be transformed into the other gradually. Note that a motion is a gradual (continuous) set of orientations and that we are here speaking of a continuous set of motions. Two motions are near only when each stage of the motion is near the corresponding stage of the other. We have twice used the idea of a map from the unit interval to something:

A more careful presentation

We define here some of the above terms more carefully.

An orientation moves all the points within a ball so that:

The unit interval is the space of reals [0, 1].

Two orientations O and P are near iff for each point x, O(x) is near P(x).

A motion is a map from the unit interval to the set of orientations such that f(0) = f(1) = the identity orientation.

Two motions M and N, are equivalent when there is a map g from the unit interval to motions, such that g(0) = M and g(1) = N. If x is in [0, 1] then g(x) is a map from [0, 1] to the set of orientations. We require that G(x, y) = g(x)(y) be a continuous function from [0, 1]×[0, 1] to the orientations.

Further result

A further result is that any motion of the ball that is equivalent to the null motion (such as a 720 degree rotation) can be extended to a finite concentric spherical region of fluid outside the ball. The fluid motion will be continuous but not rigid. The fluid motion will also be continuous with the ball and the region beyond the fluid. The final position of the fluid elements will be where they started.

Imagine concentric spheres outside our ball extending out to twice the radius. The region beyond the ball is composed of concentric spheres each of which will move rigidly with regard to itself. Consider the stages of deformation of the loop to a point. Associate a concentric sphere to each stage, inner spheres with the 720 degree rotation, and the outermost sphere with the null rotation. Each sphere now follows the rotation program prescribed by that stage of the deformation. The original homotopy required that the position be simultaneously continuous in the deformation parameter, and the “Progress of motion” parameter. That continuity assures that the fluid motions that we have just described will be continuous.

With this result we can argue that the strap is untwisted after a 720 degree rotation. (Embed the strap in the molasses.) It is not clear from this that it is twisted after 360.

Is this a proof?

Well I wouldn’t submit it to a refereed journal and I wouldn’t present it in a seminar, but I do show it to mathematical friends. I believed the proposition before I discovered the proof and even before I saw the proof using quaternions. With the quaternion proof I recognized a mathematical proof. With the above proof I feel that I understand why it is true. I also have more hope of learning whether it is true in more dimensions. P.S. It is true. See Clifford Algebras.

Well actually here is the case: It takes at least two dimensions in order to turn at all but in 2D turning 4π is not the same as turning 0π. Indeed for every n a rotation of 2πn is unique and yet brings us back to the same orientation. For more than two dimensions the situation is like 3D as described above.